The final exam will contain 15-20 questions identical to or very similar to the following. Some of these questions will be explicitly done using Excel.

1. Explain what an expectation value is and how that forms the basis for estimating statistical probabilities.

2. What is the overall value of using statistics in building and testing a model against some data set?

3. I wish to give 15% of my class and A on a midterm exam but I also want those students to get more than 87% on the midterm exam. My exam has an average score of 79%. What must the standard deviation on my exam be in order to meet my goals?

4. Review the explicit calculation of &chi²; conceptually understand expected frequencies

5. Know how to use the regression tool in Excel!

6. Know how to set up a &chi² analysis in excel.

7. Know how to use excel to do &sigma clipping

8. The Zinderland News (ZNN) has just released a story where Zindertists (a.k.a. Zinderland scientists) have reprogrammed the ZNA of Zinderbites so that Zindernoodles can grow faster. Since everyone in Zinderland understands regression. The ZNN story merely said:
   Growth = (2.0 +/- 0.5) * Zbites + 2; &sigma =0.7

   Using Zbite = 10, calculate the 95% confidence levels on Growth

9. Explain how the central limit theorem helps show the statistical power of independent and random sampling of some parent population.

10. Describe some techniques you can employ when you are working with noisy data

11. If each step I take is 2 feet and I take 1600 steps, each one in a random direction, on average, how far have I moved from my point of origin?

12. Stephanie, an expert sailor, is able to sail a specific course with an average time of 16 +/- 1 minute based on N=16 trials. Evan, a complete novice, sailed the same specific course, but due to sea sickness problems, was only able to complete N=4 trials. His average time was 20 +/- 4 minutes. Is Stephanie a significantly better sailor than Evan?

13. Explain how the D_max statistic is derived in the KS test and why the KS test is such a powerful statistical test when comparing one distribution with another.

14. On average, 4 dead Zardhogs appear every 2 miles of Texas highway. What is the probability that a Zardhog cleaning crew will have to process 6 dead Zardhogs in 1 mile of Texas Highway?

15. What assumptions need to be made to treat observed data as a Poission distribution?

16. Physically explain how the presence of a planetary atmosphere leads to a "greenhouse" effect.

17. Why does water vapour act as the primary greenhouse gas on the Earth?

18. Explain why, in the longer run (e.g. greater than 100 years) methane is likely to be the dominant greenhouse gas in the Earth's atmosphere

19. What is the principle difference between logistic growth and pure exponential growth.

20. Explain why the Lotka-Volterra model predicts a density dependent lag time between predator and prey abundance. Which reaches (predator or prey) their peak density first and why?

21. Explain how the concept of predator "handling time" acts as a feedback that can control prey density.

22. Explain some of the difficulties associated with producing an accurate estimation of the projected US population in the year 2050.

23. Explain why the assumption of a continually declining exponential growth model for the world's population may be unrealistic. Provide a conceptual example of what a more realistic model might be.

24. Review Estimation techniques and its components

25. Explain why the adult survival probability is the critical parameter that determines &lambda (the growth rate of mammalian populations

26. Explain why finite age effects (the W parameter) have such a strong effect on the crash rate (e.g. negative growth rate) of mammalian populations

27. Explain why an estimate of the demographic potential of a species, k, provides an important indicator of how habitat loss can be used to determine the decline in species population

28. In calculating the effects of habitat loss on a species, three critical variables are used: p, h and k. Explain what each of these are and which of these there is the most important to accurately measure.

29. Explain how probabilities can still be assigned to events in the case of a skewed statistical distribution in which the median is numerically less than the average value.

30. For physical events that occur in nature (e.g. floods, wind speeds, etc) explain why a skewed distribution is a better fit to such data than a normal distribution.

31. In a Weibull distribution, what does the shape parameter control?

32. For a time series analysis that shows periodic fluctuations, what basic function is used to describe those fluctuations and what are the parameters of that function?

33. Below is the time series showing the current price of crude oil.

    

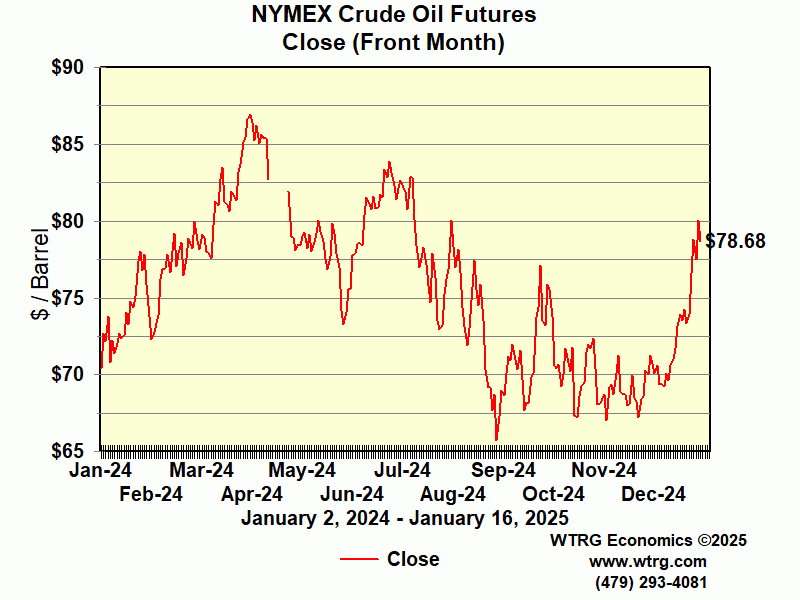
    a) Provide a qualitative description of this waveform

    b) Your boss orders you to predict the value in the year 2010 - describe your procedure for doing this

34. Explain why the detection of climate change is intrinsically difficult.

35. Explain why we know that the El Nino/La Nina cycle can't be the major driver of the observed regional climate changes, over time, in the Pacific Northwest.

36. Explain why you should always, always, always plot your data.
    
    
    
    
    
    Announcements